Ed è fatta: perivo, una ferita pesa, cader pedoni tra matti fa resa.
A sera fitta Martino depreda case, patire fa nuovi re. Patta? Fede.
venerdì 21 dicembre 2012
mercoledì 17 ottobre 2012
A volte serve una disperata voglia di vincere.
Mossa al nero. Questa posizione sembra facile, ma ci sono insidie ovunque. Prima di addentrarmi nell'analisi avverto che mi sto nascondendo dietro il cosiddetto tablebase, una macchinina magica che mi dà le risposte alle domande più improbabili. Mi limiterò ad analizzare alcune varianti (non ho ancora un'idea globale dello studio che sto facendo).
Per prima cosa bisogna accorgersi che il re bianco è in stallo e perciò è una buona idea allontanare la torre. 1...Te2 2.Rg1! Quello che il nero vuol fare è giocare Rf2 e avanzare il pedone 'f'. Bisogna stare attenti alle trappole di stallo stile "torre suicida", nella fattispecie ora 2...h2? 3.Rh1 porterebbe alla patta. In realtà ora il piano vincente è unico: bisogna portare il re in e1. 2...Re4! (non certo Re3 per lo scacco in a3). 3.Te8+ Rd3 4.Td8+ Re3!! e non 4...Rc2? 5.Ta8! patta! Il bianco non sfugge agli scacchi laterali. 5.Te8+ Rd2 6.Td8+ Re1 7.Tf8 Te4 8.Rh2! La posizione merita un diagramma.
C'è un'unica mossa che vince. 8...Rf2!! E' l'unica mossa con progresso che rende la presa in h3 inefficace (cf. sotto). Esaminiamo 9.Ta8 Te3 10.Ta1. Il piano vincente è unico.
La mossa 10...Re2? obbligherebbe il nero a riportarsi nella posizione iniziale dopo 11.Rg1 Rf3. 10...Te1! 11.Ta3 (per impedire Rf1) 11...Te8! L'unico piano vincente è allontanare la torre per disattivare il re bianco quando catturerà in h3. 12.Ta1 f3! Con la torre in e3 questa spinta non era possibile per Rxh3. Ora se 13.Rxh3 il nero vince facilmente con 13...Th8+ 14.Rg4 Rg2. 13.Ta2+ Rf1 14.Rg3. Di nuovo c'è un'unica mossa che vince.
14...h2! 15.Txh2 (15.Rxh2 Te2+) Rg1! 16.Ta2 f2 e vince.
Esaminiamo 9.Rxh3 Te3+ 10.Rh2 f3 11.Ta8. C'è un'unica mossa che vince.
Per
esempio dopo 11...Te2 sarebbe patta per 12.Rh3 Rf1 13.Rg3 f2 14.Rf3! La
torre nera deve mantenersi in e3 per parare gli scacchi laterali ed
evitare che il re bianco si attivi tramite la terza traversa. 11...Rf1! 12.Ta7 (12.Rg3 f2+!) f2 13.Ta1+ Te1 14.Ta2 Td1 con facile vittoria.
domenica 15 aprile 2012
Disperazione scacchistica
Oggi, nel corso di un torneo universitario (sette turni, cadenza 15 minuti) in cui ho fatto una prestazione magra (ottavo su venti), mi è capitata la seguente posizione disperata (io il nero):
Avvedendomi che spostare la donna non avrebbe fatto che accelerare la fine (la minaccia è Rxf5) mi sono deciso a non abbandonare e a giocare la spiritosa 1...f6, a cui è seguito 2.exf6 Rxf6 3.Nxf6 Bxf6. Ora, non so bene cosa il mio avversario abbia visto ma sta di fatto che ha giocato 4.Rxe6, sacrificando l'alfiere. E' seguito 4...Bxg5 5.Rxg6+ Ng7 6.Qe6+ Kh8 7.Qe5 Be3+ 8.Kh1 Ra7 9.Rgf6 Qa8+!
Il mio avversario si è rifiutato di coprire di donna entrando in una variante rischiosissima: 10.R1f3? Ra1+ 11.Kg2 Rg1+ 12.Kh3 Qc8 13.R6f5?? La torre sbagliata! Si priva della minaccia Rf8+. 13...Rg5!
Per il bianco non c'è più niente da fare. 14.Kh4 Rxf5 15.Rxf5 Qxf5 16.Qb8+ Kh7 17.Qb7 Bf2#
domenica 8 gennaio 2012
[Math pills] Abelian maximal subgroups
Burnside normal p-complement theorem. Let p be a prime number, G a finite group, and P a p-Sylow subgroup of G. If P is central in its normalizer then it admits a normal complement in G.
[See here]
Thompson's thesis result. Let G be a finite group admitting a fixed-point-free automorphism of prime order. Then G is nilpotent.
[See here]
I found here a nice proof of the following result using the previous two. I will write it down with some details.
Herstein Theorem. Let the finite group G admit an abelian maximal subgroup. then G is solvable.
Proof. Let M be an abelian maximal subgroup of G. Since the normal core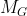 of M in G is abelian, we are reduced to show that
of M in G is abelian, we are reduced to show that 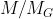 is solvable, hence we may assume that
is solvable, hence we may assume that 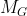 is trivial. Let p be a prime divisor of
is trivial. Let p be a prime divisor of 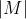 , and let P be a Sylow p-subgroup of G containing a Sylow p-subgroup of M. Suppose
, and let P be a Sylow p-subgroup of G containing a Sylow p-subgroup of M. Suppose 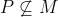 . Since P is a p-group, there exists
. Since P is a p-group, there exists 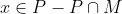 which normalizes
which normalizes  . Since M is abelian and maximal in G,
. Since M is abelian and maximal in G, 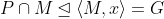 , contradicting
, contradicting 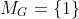 . Therefore
. Therefore 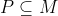 . This proves that M is a Hall subgroup of G, i.e.
. This proves that M is a Hall subgroup of G, i.e. 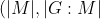=1) . By Burnside normal p-complement theorem, every Sylow p-subgroup of M admits a normal complement in G, and the intersection of these normal complements gives a normal complement N of M in G. Let
. By Burnside normal p-complement theorem, every Sylow p-subgroup of M admits a normal complement in G, and the intersection of these normal complements gives a normal complement N of M in G. Let 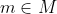 be an element of prime order. Since M centralizes m, it normalizes
be an element of prime order. Since M centralizes m, it normalizes 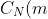) , thus
, thus 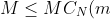 \leq G) . If
. If 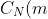 \neq \{1\}) then the maximality of M implies that
then the maximality of M implies that 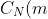=N) , i.e.
, i.e. 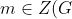) , contradicting
, contradicting 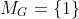 . Therefore
. Therefore 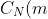=\{1\}) , so m induces a fixed-point-free automorphism of N of prime order, and by Thompson's result N is nilpotent. Since
, so m induces a fixed-point-free automorphism of N of prime order, and by Thompson's result N is nilpotent. Since  is abelian, this implies that G is solvable.
is abelian, this implies that G is solvable.
[See here]
Thompson's thesis result. Let G be a finite group admitting a fixed-point-free automorphism of prime order. Then G is nilpotent.
[See here]
I found here a nice proof of the following result using the previous two. I will write it down with some details.
Herstein Theorem. Let the finite group G admit an abelian maximal subgroup. then G is solvable.
Proof. Let M be an abelian maximal subgroup of G. Since the normal core
Iscriviti a:
Post (Atom)








